In Vivo Three-dimensional Intervertebral Kinematics of the Subaxial
Cervical Spine During Seated Axial Rotation and Lateral Bending
Via a Fluoroscopy-to-CT Registration Approach

This section was compiled by Frank M. Painter, D.C.
Send all comments or additions to:    Frankp@chiro.org

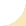
FROM:   J Biomech. 2014 (Oct 17); 47 (13): 3310–3317 ~ FULL TEXT

Amir Ahmadi, Nader Maroufi, Hamid Behtash, Hajar Zekavat, and Mohamad Parnianpour

Institute of Biomedical Engineering,
National Taiwan University,
Taipei, Taiwan, ROC.

---

---

Accurate measurement of the coupled intervertebral motions is helpful for understanding the etiology and diagnosis of relevant diseases, and for assessing the subsequent treatment. No study has reported the in vivo, dynamic and three-dimensional (3D) intervertebral motion of the cervical spine during active axial rotation (AR) and lateral bending (LB) in the sitting position. The current study fills the gap by measuring the coupled intervertebral motions of the subaxial cervical spine in ten asymptomatic young adults in an upright sitting position during active head LB and AR using a volumetric model-based 2D-to-3D registration method via biplane fluoroscopy. Subject-specific models of the individual vertebrae were derived from each subject's CT data and were registered to the fluoroscopic images for determining the 3D poses of the subaxial vertebrae that were used to obtain the intervertebral kinematics. The averaged ranges of motion to one side (ROM) during AR at C3/C4, C4/C5, C5/C6, and C6/C7 were 4.2°, 4.6°, 3.0° and 1.3°, respectively.

The corresponding values were 6.4°, 5.2°, 6.1° and 6.1° during LB. Intervertebral LB (ILB) played an important role in both AR and LB tasks of the cervical spine, experiencing greater ROM than intervertebral AR (IAR) (ratio of coupled motion (IAR/ILB): 0.23-0.75 in LB, 0.34-0.95 in AR). Compared to the AR task, the ranges of ILB during the LB task were significantly greater at C5/6 (p=0.008) and C6/7 (p=0.001) but the range of IAR was significantly smaller at C4/5 (p=0.02), leading to significantly smaller ratios of coupled motions at C4/5 (p=0.0013), C5/6 (p<0.001) and C6/7 (p=0.0037). The observed coupling characteristics of the intervertebral kinematics were different from those in previous studies under discrete static conditions in a supine position without weight-bearing, suggesting that the testing conditions likely affect the kinematics of the subaxial cervical spine.

While C1 and C2 were not included owing to technical limitations, the current results nonetheless provide baseline data of the intervertebral motion of the subaxial cervical spine in asymptomatic young subjects under physiological conditions, which may be helpful for further investigations into spine biomechanics.

KEYWORDS:   Cervical spine; Computed tomography; Coupled motion; Fluoroscopy; Image registration; Intervertebral motion; Kinematics; Weight-bearing

---

From the FULL TEXT Article:

Introduction

The cervical spine supports the weight of the head and facilitates its motion during activities of daily living via six degrees-of- freedom motions of all motion segments. The coupling of the six components of the intervertebral motion during a head movement has been related to the geometry of the vertebrae, constraints of the soft tissues, the curvature of the spine and the control of the surrounding muscles (Kapandji, 1970; Milne, 1993; Penning, 1988; White and Panjabi, 1990). Accurate measurement of the coupled intervertebral motion patterns is helpful for characterizing the geometric changes of the intervertebral discs (Wang et al., 2011), for manual therapists in managing relevant clinical problems (Cook et al., 2006), for assessing the effects of surgical fusion on motions of adjacent vertebrae (Fuller et al., 1998; Schwab et al., 2006), and for evaluating various surgical approaches (Liu et al., 2007; McDonald et al., 2014; Rabin et al., 2007).

Several methods have been developed for measuring the three-dimensional (3D) kinematics of the cervical spine in vivo (Ishii et al., 2004a; Mimura et al., 1989; Salem et al., 2013; Wang et al., 2005), but not many allow for non-invasive measurement of the 3D, dynamic intervertebral motion under physiological weight-bearing conditions (Anderst et al., 2011; Lin et al., 2013). Somebody surface measurement systems measure the relative movement between the head and trunk to describe the coupled motions of the cervical spine as a whole (Ngan et al., 2005; Wang et al., 2005). Others investigate the coupled intervertebral motion patterns using biplane radiography (Iai et al., 1993; Mimura et al., 1989) but with limited reliability mainly as a result of the high measurement variations in the digitization procedure (Bogduk and Mercer,2000). For more accurate descriptions of the 3D intervertebral motions, methods based on computed tomography (CT) (Salem et al.,2013) and magnetic resonance imaging (MRI)have been proposed (Ishii et al., 2006, 2004a, 2004b). With these approaches, the subjects had to be tested in a supine position and only quasi-static measurements could be made. Therefore, measurements of intervertebral motions during dynamic, physiological, weight-bearing motions were not possible. The quasi-static, coupled motions of the cervical vertebrae quantified by these methods, such as the ratio of the coupled motion at full axial rotation (AR), remain controversial (Salem et al., 2013).

Recently, model-based 2D-to-3D registration methods via single-plane fluoroscopy (Lin et al., 2013) or high-speed biplane radiography (Anderst et al., 2011; Haque et al., 2012; McDonald et al., 2010) have been developed for non-invasive measurement of 3D, dynamic intervertebral kinematics of the cervical spine, and have been used to study intervertebral motions in sitting positions during neck flexion and extension in healthy subjects (Anderst et al., 2013a, 2013b) and AR in patients (McDonald et al., 2014). To the best knowledge of the authors, similar studies for comparing lateral bending (LB) and AR have not been reported. Therefore, the purpose of this study was to quantify the in vivo, dynamic, 3D intervertebral kinematics of the subaxial cervical spine in healthy young subjects during active LB and AR in a sitting position using a volumetric model-based 2D-to-3D registration method based on biplane fluoroscopy. It was hypothesized that the ranges of the intervertebral motion and the coupled patterns of the motion components would be significantl different between AR and LB tasks.

---

Materials and methods

      Subjects and data acquisition

Figure 1 Figure 2

Ten healthy young adults (four females and six males; age: 22.6 ± 2.6 years), without any neck or shoulder symptoms or history of cervical disorders or trauma, participated in this study with written informed consent approved by the Institutional Research Board. Each subject received a CT scan (Light Speed VCT, GE Healthcare, Little Chalfont,UK) over a range from the occiput to the C7-T1 junction with a voxel size of 0.4 x 0.4 x 0.625 mm³. The CT data were used to construct subject-specific surface (Figure 1a) and volumetric models (Fig. 1b) of the vertebrae via segmentation and reconstruction processes using a software package (Amira, Visage Imaging Inc., Germany)(Lin et al.,2013).

The subjects were asked to perform active AR and LB tasks while sitting on a chair with the trunk stabilized and under the surveillance of a biplane fluoroscope (Allura Xper FD10/10,Philips Medical Systems, Netherlands)so that two sequences of fluoroscopic images of the cervical spine could be obtained at a sampling rate of 15 frames/s (exposuretime: 3ms/frame; peak voltage: 60kVp; mean tube current: 138.1mA)(Figure 2). The two image sequences were synchornized with a constant time difference of 1/30 s to avoid the so-called ‘Compton Scattering’ problem caused by simultaneous exposures. Data of a singletrial for A Rand LB, each lasting about 11.2s and 10.2s, respectively, were collected for each subject. During AR, each subject turned (or rotated) the head to the left side until the limit of a maximum stretch without feeling uncomfortable, followed by a rotation of the head towards the rights ide, where after the head returned to the neutral position. During LB, the subject tilted (or bent) the head toward the left shoulder until the limit of a maximum stretch was achieved, followed by tilting the head towards the contralateral shoulder to achieve the maximum neck stretch.

Finally, the head returned to the neutral position. Before data collection the subject was asked to practise AR and LB several times under the supervision of a physical therapist until the subject was able to perform the primary motion in the required plane (i.e., frontal plane for LB and transverse plane for AR) without any guidance during the tests. Two digital cameras (HDR-SR1, Sony, Japan) were placed on the front and right side of the subject to monitor the motions of the head/cervical spine. A trial would be excluded from analysis if the motions were not in the required planes as indicated by the videorecordings. The motion of the head relative to the trunk was also measured using skin-marker based stereophotogrammetry (VICON, Oxford Metrics, UK).

      Model-based registration

For registering the CT data to the fluoroscopy images, each X-ray tube/detector pair of the biplane fluoroscopy system was described as an ideal perspective projection model (Fig. 2b). Prior to data collection, the intrinsic parameters for each projection model, and the extrinsic parameters for describing the spatial transformation of the two projection models, were determined (Fig. 2b). The intrinsic parameters were obtained through a calibration procedure using a purpose-built calibration box consisting of a reseau plate and a star-shaped plate with lead markers (Lu et al., 2008). Another calibration procedure using a calibration object with several known positioned lead markers was also performed to determine the extrinsic parameters (Kaptein et al., 2011).

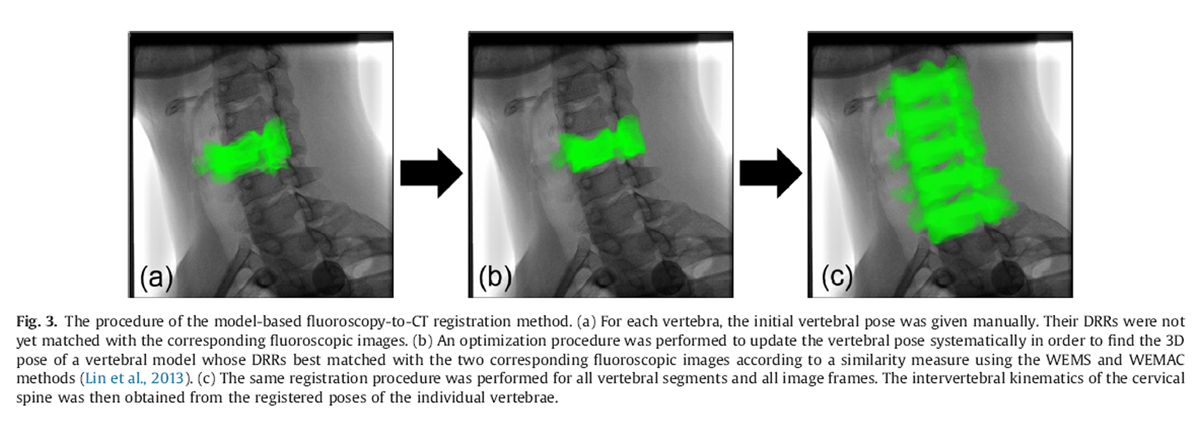
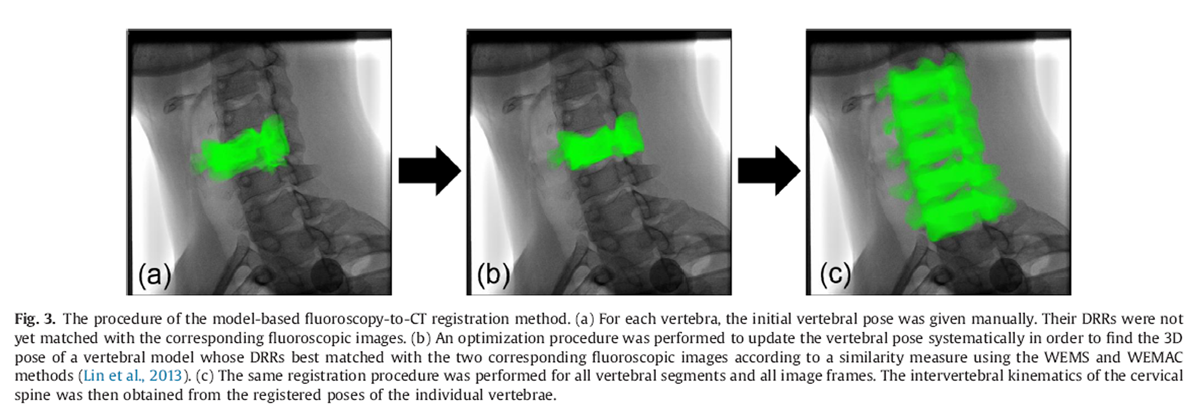
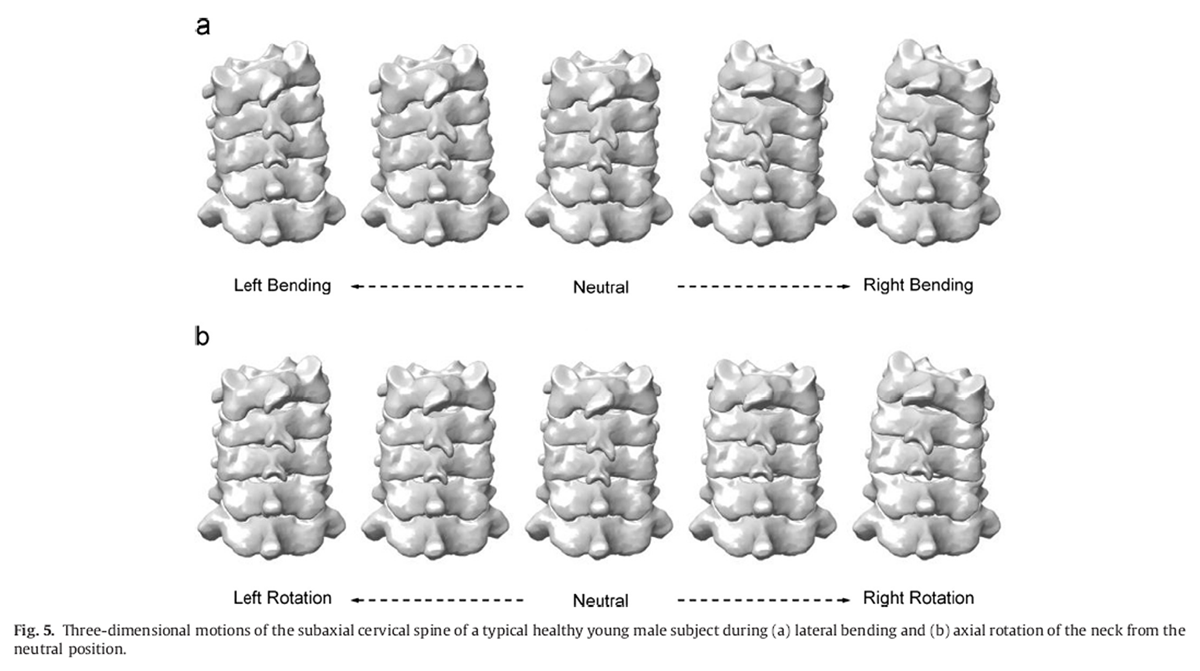
Figure 3 Figure 4 Figure 5

Given a volumetric vertebral model in space, a digitally reconstructed radiograph (DRR) was produced for each projection model of the fluoroscopy system by casting rays from the X-ray point source. Each of these rays went through a number of voxels of the volume, the attenuation coefficients of which were integrated along the ray and projected onto the image plane to obtain the DRR using a ray-tracing method with tri-linear interpolation (Hadwiger et al., 2006). For each image frame, an optimization procedure was used to find the 3D pose of a vertebral model whose DRRs best matched the two corresponding fluoroscopic images according to a similarity measure called the weighted edge-matching score (WEMS) (Lin et al., 2014; Tsaietal., 2010) (Figure 3). The initial poses of the volumetric models of the vertebrae (Fig.1b) for the optimization procedure of the registration were obtained manually using the corresponding surface models (Fig.1a) with the assistance of a graphical user interface (GUI) program developed in Matlab(Mathworks Inc., USA) (Figure 4). The registration process was performed for all image frames of the tested weight-bearing activities (Figure 5).

If the images of a vertebra in one fluoroscopyplane became unavailable (e.g., out of field-of-view or completely obscured by the head), the WEMS with anti-collision constraints method (WEMAC), a single-plane fluoroscopy-based method, was used to register the vertebra using the images from the other fluoroscopy plane (Lin et al., 2013). Such conditions affected only the C3 around the extremes of the head movement in a small proportion of trials when the C3 was obscured by the mandible or the occipital. The registration method using biplane fluoroscopy has been shown to be of high accuracy, with means (standard deviations)of errors less than 0.17 (0.34) mm and 0.09° (0.60°) for translations and rotations, respectively (Lin et al., 2014). The WEMAC method was also shown to have sub-millimeter and sub-degree accuracy, which was considered accurate for 3D intervertebral kinematic measurements (Lin et al., 2013). The registered 3D poses of the vertebrae were used for subsequent calculation of kinematic variables without smoothing or filtering.

      Kinematic analysis

Each vertebral model was embedded with an anatomical coordinate system (ACS) defined using an atomical features of the vertebra as described by Ishii et al. (2006). The ACS originated at the most posterio-inferior point of the vertebral body in the mid-sagittal plane, with the positive x-axis directed anteriorly, the positive y-axis directed superiorly and the positive z-axis determined as the cross-product of the x- and y-axes.

With the registered poses of the vertebrae, the intervertebral joint angles were determined as the rotation of the ACS of a vertebra relat ive to that of the subjacent vertebra using a z–y–x (or flexion/extension–axial rotation–lateral bending) sequence following Ishii et al.(2006). Similarly, the intervertebral translations were defined as the relative displacement of the origins (Ishii et al., 2006). The ranges of motion to one side (ROM) for each of the six components of a motion segment were calculated as the differences of intervertebral poses between the neutral and extreme positions, i.e., the maximum displacements achieved to the right and to the left. For comparisons between motion segments and between studies, the ROM values for each motion segment were normalized to the sum of the values of all subaxial motion segments. The coupling of the intervertebral AR (IAR) and intervertebral LB (ILB) during the test activities was described by the ratio of the ROMs of the IAR and ILB, i.e., IAR/ILB, called the ratio of the coupled motion (RCM).

      Statistical analysis

The means and standard deviations (SD) of each of the six intervertebral kinematic components of all subjects were obtained at 5° increments of ILB angles of C3/C7 during LB and at 2° increments of IAR angles of C3/C7 during AR. Means and standard deviations (SD)of the ROM and RCM for all subaxial motion segments across all subjects were also calculated. Paired t-tests were performed to test the differences in the ROM and RCM between the left and right side for all subaxial levels. Paired t-tests were also performed to test the differences in ILB, IAR and RCM between LB and AR tasks for all subaxial levels. For all tests, a significance level of 0.05 was selected. All statistical analyses were performed using SPSS Version 11.0 (SPSS Inc., Chicago,USA).

---

Results

Figure 6 Table 1 Table 4

No significant differences in the ROM and RCM were found between the motions to the left and to the right (ROM: p > 0.17; RCM: p > 0.21). Therefore, the values to both sides were pooled for each variable. Between the AR and LB tasks, the patterns of the intervertebral motion components and their coupling characteristics were largely different(Figure 6).

During the AR task, the means (SD) of the maximum IAR to one side were 4.2° (1.3°) at C3/C4, 4.6 (1.3°) at C4/C5, 3.0° (1.0°) at C5/C6 and 1.3° (0.8°) at C6/C7, with considerable ipsilateral coupled ILB (Table 1). The means (SD) of the RCM were 0.75 (0.26)at C3/C4, 0.95 (0.19) at C4/C5, 0.60 (0.15) at C5/C6 and 0.34 (0.22) at C6/C7 (Table 4). The coupled intervertebral flexion/extension (IFE) motions were relatively variable among subjects (Table 1). Contralateral intervertebral translations of less than 1.1mm, i.e., translation to the right during left AR and vice versa, were also found for all segments. The translations in the other two directions were smaller (<0.2 mm) (Table 1).

For the LB task, the means (SD)of the maximum ILB to one side were 6.4° (2.3°) at C3/C4, 5.2° (1.4°) at C4/C5, 6.1° (1.8°) at C5/C6 and 6.1° (1.8°) at C6/C7 with considerable ipsilateral coupled IAR (Table 1). The coupled IAR during LB gave means (SD) of the RCM of 0.70 (0.20), 0.75 (0.20), 0.47 (0.12) and 0.23 (0.19), respectively (Table 4). Compared to the AR task, the ranges of ILB during the LB task were significantly greater at C5/6(p = 0.008) and C6/7 (p = 0.001) but the range of IAR was significantly smaller at C4/5 (p = 0.02). The RCM during LB was significantly smaller than those during AR for levels of C4/C5 (p = 0.0013), C5/C6 (p = 0.001) and C6/C7 (p = 0.0037). Coupled intervertebral flexion and contralateral intervertebral translations of less than 1mm, i.e., translation to the right during left LB and vice versa, were found in all segments during the LB task (Table 1). The intervertebral translations in the other two directions were smaller (<0.4mm) (Table 1).

---

Discussion

Few data of the in vivo intervertebral kinematics of the cervical spine during LB and AR tasks under physiological weight-bearing conditions have been reported mainly owing to measurement difficulties. The current study successfully bridged the gap by integrating the vertebral CT volumetric data and real-time fluoroscopic imaging via a fluoroscopy-to-CT registration approach. The current results accurately revealed the coupled characteristics of the cervical intervertebral motions during the tested activities.

Table 2

During the active AR task, the segmental contributions to the total subaxial ROM were the greatest at C4/C5, followed by C3/C4 and C5/C6, with C6/C7 being the smallest, in agreement with most of the previous studies (Ishii et al., 2004a; Panjabi et al., 2001; Penning and Wilmink, 1987; Salemetal., 2013; Wenetal., 1993). However, the quantitative contributions appeared to be controversial in the literature (Table 2). The current results showed that the middle segments contributed about 67% of the total subaxial ROM and that C6/C7 contributed about 10%, which is closer to the data reportedby Ishii et al. (2004a). Other studies reported smaller contributions in the middle segments but a greater contribution at C6/C7. The ROM of the ILB secondary to the primary motion was found to be greater than the primary IAR for all segmentsas indicated by RCM values of less than 1, also in qualitative agreement with Ishii et al. (2004a). Similar patterns of coupled intervertebral ROM were found by Mimura et al. (1989)), exceptfor C6/C7 where the ROM of the secondary ILB was smaller than those of the primary IAR. These in vivo results were in contrast to results reported in a previous in vitro study (Wen et al.,1993) and an in vivo study during passive AR (Salem et al.,2013), where the ROM of the secondary ILB was smaller than the primary ROM (i.e., RCM41). In the sagittal plane, no or minimum coupled extension or flexion was found in the current study (Table 1), which was in contrast to previous in vivo studies reporting that coupled extension occurred in the middle levels, and that coupled flexion occurred in the lower levels (Ishii et al., 2004a; Salem et al.,2013).

The differences in the ROM and the coupling patterns of the subaxial segments noted between the current and previous studies on young healthy subjects may be explained by factors such as the external loading condition, posture and muscle action (i.e., active vs. passive motion). Previous studies have demonstrated that intervertebral kinematics would be altered by different cervical curvatures (Miyazaki et al., 2008; Takeshima et al., 2002), different head postures (Edmondston et al., 2005), different involvement of muscle actions (Dvorak et al., 1988) and different loading conditions (Voo et al., 1998). Generally, a passively rotated spine would produce greater ROM in all subaxial motion segments than an actively rotated one, as indicated by the differences in the sum of ROM between the current study and Salem et al.(2013) (Table 2). The similarities in the IAR and coupled ILB between the current study and Ishii et al. (2004a) may suggest that the effects of tested position (supine vs.upright) and loading conditions (static vs.dynamic) during active AR were mainly on the coupled motions in the sagittal plane (Ishii et al., 2004a; Salem et al., 2013). Other factors, such as the rotation sequence used for the ROM calculation, may also contribute to the differences in the ROM values reported in different studies (Panjabi et al.,1981).

Table 3

During the active LB task, the ranges of ILB, segmental contributions to the total subaxial ROM and the coupling with IAR were clearly different from those during active AR. The segmental contributions to the total subaxial ROM were similar among all subaxial motion segments. This was in contrast to Panjabi et al. (2001) (i.e., greater in middle levels) and Ishii et al.(2006)) (i.e., greater in lower levels) (Table 3). The results were also different compared to those during the AR task in which contributions were greatest at C4/C5 and smallest at C6/C7 (Table 2). The associated RCM was significantly smaller than that during the AR task, except at C3/C4, owing to the differences in the ILB and IAR. The RCM during the LB task in the current study was also greater compared to that of previous studies (Ishii et al., 2006; Wen et al., 1993) (Table 4). These differences were also likely to be related to the posture and loading conditions including muscle actions used in the studies. In the neutral position during sitting, the gravitational force on the head tended to pass through the motion segments, thus reducing the bending moments and axial torques at the motion segments. However, increased bending moments due to gravity of the head would be generated during active LB. The further the head is displaced from the base of the cervical spine (i.e., C7), the greater the counter-acting bending moments that would be needed at the motion segments, with different effects on the intervertebral kinematics. This more physiological phenomenon could not be observed at discrete static LB angles in a supine position without weight-bearing.

The current study was limited to the kinematics of the mid and lower segments of the cervical spine in young asymptomatic adults during AR and LB. The motions of the C1 and C2 segments were not included because of the limited field-of-view of the biplane fluoroscopy system used, as well as the obstruction of the images of C1 and C2 by th emandibular and occipital bones in certain postures. This limitation could be addressed by using larger fluoroscopy detectors in further studies. In order to minimize radiation dosage, a single trial per motion was collected in the current study. Further study on the repeatability of the intervertebral motions of the cervical spine may be feasible when a low-dose biplane fluoroscopy system becomes available. Further study on other age groups will also be needed for clarifying the age effects on the cervical intervertebral motion characteristics.

In conclusion, in vivo, 3D coupled motions of the subaxial cervical spine during dynamic LB and AR under physiological weight-bearing conditions were accurately measured and documented for the first time in the literature using the volumetric model-based 2D-to-3D registration method via biplane fluoroscopy. Intervertebral LB played an important role in both LB and AR of the cervical spine, experiencing greater ranges of motion than IAR. The observed coupling characteristics of the intervertebral kinematics were different from those in previous studies under discrete static conditions in a supine position without weight-bearing, suggesting that the testing conditions likely affect the kinematics of the subaxial cervical spine.

---

References:

• Anderst, W.J., Baillargeon, E., Donaldson, W.F., Lee, J.Y., Kang, J.D., 2011.
  Validation of a noninvasive technique to precisely measure in vivo three-dimensional cervical spine movement.
  Spine 36, E393–E400

• Anderst, W.J., Donaldson, W.F., Lee, J.Y., Kang, J.D., 2013a.
  Cervical spine intervertebral kinematics with respect to the head are different during flexion and extension motions.
  J.Biomech. 46, 1471–1475.

• Anderst, W.J., Lee, J.Y., Donaldson 3rd, W.F., Kang, J.D., 2013b.
  Six-degrees-of-freedom cervical spine range of motion during dynamic flexion-extension
  after single-level anterior arthrodesis:comparison with asymptomatic control subjects.
  J. Bone Joint Surg.(American) 95, 497–506.

• Bogduk, N., Mercer, S.,2000.
  Biomechanics of the cervical spine. I: Normal kinematics.
  Clin. Biomech. 15, 633–648.

• Cook, C., Hegedus, E., Showalter, C., Sizer Jr, P.S.,2006.
  Coupling behavior of the cervical spine: a systematic review of the literature.
  J. Manipulative Physiol. Ther. 29, 570–575.

• Dvorak, J., Froechlich, D., Penning, L., Baumgartner, H., Panjabi, M.M., 1988.
  Functional radiographic diagnosis of the cervical spine: flexion/extension.
  Spine 13, 748–755.

• Edmondston, S.J., Henne, S.E., Loh, W., Østvold, E., 2005.
  Influence of cranio-cervical posture on three-dimensional motion of the cervical spine.
  Man. Ther.10, 44–51.

• Fuller, D.A., Kirkpatrick, J.S., Emery, S.E., Wilber, R.G., Davy, D.T., 1998.
  A kinematic study of the cervical spine before and after segmental arthrodesis.
  Spine 23, 1649–1656.

• Hadwiger, M., Kniss, J.M., Rezk-salama, C., Weiskopf, D., Engel, K., 2006.
  Real-time Volume Graphics.
  A.K. Peters, Ltd.

• Anderst, W., Tashman, S., Marai, G.E., 2012.
  Hierarchical model-based tracking of cervical vertebrae from dynamic biplane radiographs.
  Med.Eng. Phys..

• Iai, H., Moriya, H., Goto, S., Takahashi, K., Yamagata, M., Tamaki, T., 1993.
  Three-dimensional motion analysis of the upper cervical spine during axial rotation.
  Spine 18, 2388–2392.

• Ishii, T., Mukai, Y., Hosono, N., Sakaura, H., Fujii, R., Nakajima, Y., Tamura, S., Iwasaki, M., Yoshikawa, H., Sugamoto, K., 2006.
  Kinematics of the cervical spine in lateral bending: in vivo three-dimensional analysis.
  Spine 31, 155–160.

• Ishii, T., Mukai, Y., Hosono, N., Sakaura, H., Fujii, R., Nakajima, Y., Tamura, S., Sugamoto, K., Yoshikawa, H., 2004a.
  Kinematics of the subaxial cervical spine in rotation in vivo three-dimensional analysis.
  Spine 29, 2826–2831.

• Ishii, T., Mukai, Y., Hosono, N., Sakaura, H., Nakajima, Y., Sato, Y., Sugamoto, K., Yoshikawa, H., 2004b.
  Kinematics of the upper cervical spine in rotation: in vivo three-dimensional analysis.
  Spine 29, E139–E144.

• Kapandji, I.A., 1970.
  The physiology of the joints: annotated diagrams of the mechanics of the human joints.
  The Trunk and the Vertebral Column, Vol.3.
  Churchill Livingstone.

• Kaptein, B.L., Shelburne, K.B., Torry, M.R., ErikGiphart, J., 2011.
  A comparison of calibration methods for stereo fluoroscopic imaging systems.
  J. Biomech. 44, 2511–2515.

• Lin, C.-C., Lu, T.-W., Shih, T.-F., Tsai, T.-Y., Wang, T.-M., Hsu, S.-J., 2013.
  Intervertebral anticollision constraints improve out-of-plane translation accuracy of a single-plane fluoroscopy-to-CT registration method for measuring spinal motion.
  Med. Phys. 40, 031912.

• Lin, C.-C., Lu, T.-W., Wang, T.-M., Hsu, C.-Y., Shih, T.-F., 2014.
  Comparisons of surface vs. volumetric model-based registration methods using single-plane vs. bi- plane fluoroscopy in measuring spinal kinematics.
  Med. Eng. Phys. 36, 267–274.

• Liu, F., Cheng, J., Komistek, R.D., Mahfouz, M.R., Sharma, A., 2007.
  In vivo evaluation of dynamic characteristics of the normal, fused, and discreplacement cervical spines.
  Spine 32, 2578–2584.

• Lu, T.W., Tsai, T.Y., Kuo, M.Y., Hsu, H.C., Chen, H.L., 2008.
  In vivo three-dimensional kinematics of the normal knee during active extension under unloaded and loaded conditions using single-plane fluoroscopy.
  Med. Eng. Phys. 30, 1004–1012.

• McDonald, C.P., Bachison, C.C., Chang, V., Bartol, S.W., Bey, M.J., 2010.
  Three-dimensional dynamic in vivo motion of the cervical spine: assessment of measurement accuracy and preliminar findings.
  Spine J. 10, 497–504.

• McDonald, C.P., Chang, V., McDonald, M., Ramo, N., Bey, M.J., Bartol, S.,2014.
  Three-dimensional motion analysis of the cervical spine for comparison of anterior cervical decompression and fusion versus artificial discreplacement in 17 patients.
  J. Neurosurg.: Spine 20, 245–255.

• Milne, N., 1993.
  Composite motion in cervical disc segments.
  Clin. Biomech. 8, 193–202.

• Mimura, M., Moriya, H., Watanabe, T., Takahashi, K., Yamagata, M., Tamaki, T., 1989.
  Three-dimensional motion analysis of the cervical spine with special reference to the axial rotation.
  Spine 14, 1135–1139.

• Miyazaki, M., Hymanson, H.J., Morishita, Y., He, W., Zhang, H., Wu, G., Kong, M.H., Tsumura, H., Wang, J.C., 2008.
  Kinematic analysis of the relationship between sagittal alignment and disc degeneration in the cervical spine.
  Spine 33, E870–E876.

• Ngan, J.M.W., Chow, D.H.K., Holmes, A.D., 2005.
  The kinematics and intra- and inter-therapist consistencies of lower cervical rotational manipulation.
  Med. Eng. Phys. 27, 395–401.

• Panjabi, M.M., Crisco, J.J., Vasavada, A., Oda, T., Cholewicki, J., Nibu, K., Shin, E., 2001.
  Mechanical properties of the human cervical spine as shown by three-dimensional load–displacement curves.
  Spine 26, 2692–2700.

• Panjabi, M.M., Krag, M.H., Goel, V.K., 1981.
  A technique for measurement and description of three-dimensional six degree-of-freedom motion of a body joint with an application to the human spine.
  J. Biomech. 14, 447–460.

• Penning, L., 1988.
  Differences in anatomy, motion, development and aging of the upper and lower cervical disk segments.
  Clin. Biomech. 3, 37–47.

• Penning, L., Wilmink, J.T., 1987.
  Rotation of the cervical spine.
  ACT study in normal subjects.
  Spine 12, 732–738.

• Rabin, D., Pickett, G.E., Bisnaire, L., Duggal, N., 2007.
  The kinematics of anterior cervical discectomy and fusion ver6sus artificial cervical disc: a pilot study.
  Neurosurgery 61 (ONS-100-ONS-104).

• Salem, W., Lenders, C., Mathieu, J., Hermanus, N., Klein, P., 2013.
  
  In vivo three-dimensional kinematics of the cervical spine during maximal axial rotation.
  Man. Ther.18,339–344.

• Schwab, J.S., DiAngelo, D.J., Foley, K.T., 2006.
  Motion compensation associated with single-level cervical fusion: where does the lost motion go?
  Spine 31, 2439–2448.

• Takeshima, T., Omokawa, S., Takaoka, T., Araki, M., Ueda, Y., Takakura, Y., 2002.
  Sagittal Alignment of Cervical Flexion and Extension: lateral Radiographic Analysis.
  Spine 27, E348–E355.

• Tsai, T.Y., Lu, T.W., Chen, C.M., Kuo, M.Y., Hsu, H.C., 2010.
  A volumetric model-based 2D to 3D registration method for measuring kinematics of natural knees with single-plane fluoroscopy.
  Med.Phys. 37, 1273–1284.

• Voo, L.M., Pintar, F.A., Yoganandan, N., Liu, Y.K., 1998.
  Static and dynamic bending responses of the human cervical spine.
  J. Biomech. Eng. 120, 693–696.

• Wang, S., Xia, Q., Passias, P., Li, W., Wood, K., Li, G., 2011.
  How does lumbar degenerative disc disease affect the disc deformation at the cephalic levels in vivo?
  Spine 36, E574–E581.

• Wang,S.F., Teng, C.C., Lin, K.H., 2005.
  Measurement of cervical range of motion pattern during cyclic neck movement by an ultrasound-based motion system.
  Man. Ther. 10,68–72.

• Wen, N., Lavaste, F., Santin, J.J., Lassau, J.P., 1993.
  Three-dimensional biomechanical properties of the human cervical spine in vitro.
  Eur.Spine J. 2,2–11.

• White, A.,Panjabi,M.,1990.
  Clinical Biomechanics of the Spine.
  Lippincott Philadelphia

Return to RADIOLOGY

Since 1-232019